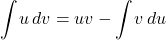3.1 Integration by Parts
Learning Objectives
- Recognize when to use integration by parts.
- Use the integration-by-parts formula to evaluate indefinite integrals.
- Use the integration-by-parts formula for definite integrals.
By now we have a fairly thorough procedure for how to evaluate many basic integrals. However, although we can integrate ![]() by using the substitution,
by using the substitution, ![]() something as simple looking as
something as simple looking as ![]() defies us. Although there is no product rule for integration, there is a technique based on the product rule for differentiation that allows us to exchange one integral for another. We call this technique integration by parts.
defies us. Although there is no product rule for integration, there is a technique based on the product rule for differentiation that allows us to exchange one integral for another. We call this technique integration by parts.
The Integration-by-Parts Formula
If ![]() then by using the product rule, we obtain
then by using the product rule, we obtain ![]()
We rewrite this formula as ![]() and then integrate both sides of the last equation:
and then integrate both sides of the last equation:
![]()
Since ![]() is an antiderivative of
is an antiderivative of ![]() , we have
, we have
![]()
By making the substitutions ![]() and
and ![]() which in turn make
which in turn make ![]() and
and ![]() we have the more compact form
we have the more compact form
![]()
Integration by Parts
Let ![]() and
and ![]() be functions with continuous derivatives. Then, the integration-by-parts formula for the integral involving these two functions is:
be functions with continuous derivatives. Then, the integration-by-parts formula for the integral involving these two functions is:
The advantage of using the integration-by-parts formula is that we can exchange one integral for another, possibly easier, integral. The following example illustrates its use.
Using Integration by Parts
Use integration by parts with ![]() and
and ![]() to evaluate
to evaluate ![]()
Solution
By choosing ![]() we have
we have ![]() Since
Since ![]() we can take
we can take ![]() to be any antiderivative of
to be any antiderivative of ![]() , and the simplest choice would be
, and the simplest choice would be ![]() It is handy to keep track of these values as follows:
It is handy to keep track of these values as follows:
![Rendered by QuickLaTeX.com \ds \begin{array}{ccccccc}\hfill u&\ds =\hfill &\ds x\hfill &\ds &\ds \hfill dv&\ds =\hfill &\ds \text{sin}\phantom{\rule{0.1em}{0ex}}(x)\phantom{\rule{0.2em}{0ex}}\,dx \hfill \\[3mm]\ds \hfill du&\ds =\hfill &\ds 1\,dx \hfill &\ds &\ds \hfill v&\ds =\hfill &\ds - \text{cos}\phantom{\rule{0.1em}{0ex}}(x).\hfill \end{array}](https://pressbooks.openedmb.ca/app/uploads/quicklatex/quicklatex.com-ac340d09d550908d0835954ed574c033_l3.png)
Applying the integration-by-parts formula (*) results in
![Rendered by QuickLaTeX.com \ds \begin{array}{cccc}\hfill \ds\int x\,\phantom{\rule{0.1em}{0ex}}\text{sin}\phantom{\rule{0.1em}{0ex}}(x)\phantom{\rule{0.2em}{0ex}}\,dx &\ds =\left(x\right)\left( - \text{cos}\phantom{\rule{0.1em}{0ex}}(x)\right)-\int \left( - \text{cos}\phantom{\rule{0.1em}{0ex}}(x)\right)\left(1\,dx \right)\hfill &\ds &\ds \text{substitute}\hfill \\[5mm]\ds &\ds = - x\phantom{\rule{0.1em}{0ex}}\text{cos}\phantom{\rule{0.1em}{0ex}}(x)+\int \text{cos}\phantom{\rule{0.1em}{0ex}}(x)\phantom{\rule{0.2em}{0ex}}\,dx \hfill &\ds &\ds \text{simplify}\hfill \\[5mm]\ds &\ds = - x\phantom{\rule{0.1em}{0ex}}\text{cos}\phantom{\rule{0.1em}{0ex}}(x)+\text{sin}\phantom{\rule{0.1em}{0ex}}(x)+C.\hfill &\ds &\ds \text{integrate} \cos(x)\hfill \end{array}](https://pressbooks.openedmb.ca/app/uploads/quicklatex/quicklatex.com-b78fb4ef871402e33853841b62155c6d_l3.png)
Analysis
At this point, there are probably a few items that need clarification. First of all, you may be curious about what would have happened if we had chosen ![]() and
and ![]() If we had done so, then we would have
If we had done so, then we would have ![]() and
and ![]() Thus, after applying integration by parts, we would get
Thus, after applying integration by parts, we would get ![]() Unfortunately, with the new integral, we are in no better position than before. It is important to keep in mind that when we apply integration by parts, we may need to try several choices for
Unfortunately, with the new integral, we are in no better position than before. It is important to keep in mind that when we apply integration by parts, we may need to try several choices for ![]() and
and ![]() before finding a choice that works.
before finding a choice that works.
Second, you may wonder why, when we find ![]() as an antiderivative of
as an antiderivative of ![]() we do not use
we do not use ![]() To see that it makes no difference, we can rework the problem using
To see that it makes no difference, we can rework the problem using ![]()
![Rendered by QuickLaTeX.com \ds \begin{array}{cc}\ds {\int }^{\phantom{\rule{0.2em}{0ex}}}x\,\phantom{\rule{0.1em}{0ex}}\text{sin}\phantom{\rule{0.1em}{0ex}}(x)\phantom{\rule{0.2em}{0ex}}\,dx \hfill &\ds =\left(x\right)\left( - \text{cos}\phantom{\rule{0.1em}{0ex}}(x)+K\right)-{\int }^{\phantom{\rule{0.2em}{0ex}}}\left( - \text{cos}\phantom{\rule{0.1em}{0ex}}(x)+K\right)\left(1\,dx \right)\hfill \\[5mm]\ds \hfill &\ds = - x\phantom{\rule{0.1em}{0ex}}\text{cos}\phantom{\rule{0.1em}{0ex}}(x)+Kx+{\int }^{\phantom{\rule{0.2em}{0ex}}}\text{cos}\phantom{\rule{0.1em}{0ex}}(x)\phantom{\rule{0.2em}{0ex}}\,dx -{\int }^{\phantom{\rule{0.2em}{0ex}}}K\,dx \hfill \\[5mm]\ds \hfill &\ds = - x\phantom{\rule{0.1em}{0ex}}\text{cos}\phantom{\rule{0.1em}{0ex}}(x)\phantom{\rule{0.2em}{0ex}}+Kx+\text{sin}\phantom{\rule{0.1em}{0ex}}(x)-Kx+C\hfill \\[5mm]\ds \hfill &\ds = - x\phantom{\rule{0.1em}{0ex}}\text{cos}\phantom{\rule{0.1em}{0ex}}(x)+\text{sin}\phantom{\rule{0.1em}{0ex}}(x)+C.\hfill \end{array}](https://pressbooks.openedmb.ca/app/uploads/quicklatex/quicklatex.com-7c5f31cb3598f1475b026dec601ce37f_l3.png)
As you can see, it makes no difference in the final solution.
Last, we can verify that our antiderivative is correct by differentiating ![]()
![Rendered by QuickLaTeX.com \ds \begin{array}{cc}\ds \frac{d}{\,dx }\left( - x\phantom{\rule{0.1em}{0ex}}\text{cos}\phantom{\rule{0.1em}{0ex}}(x)+\text{sin}\phantom{\rule{0.1em}{0ex}}(x)+C\right)\hfill &\ds =\left(-1\right)\text{cos}\phantom{\rule{0.1em}{0ex}}(x)+\left( - x\right)\left( - \text{sin}\phantom{\rule{0.1em}{0ex}}(x)\right)+\text{cos}\phantom{\rule{0.1em}{0ex}}(x)\hfill \\[5mm]\ds \hfill &\ds =x\phantom{\rule{0.1em}{0ex}}\text{sin}\phantom{\rule{0.1em}{0ex}}(x).\hfill \end{array}](https://pressbooks.openedmb.ca/app/uploads/quicklatex/quicklatex.com-3c94a3459f0d68e05a1f26ea874d84cd_l3.png)
Therefore, the answer we obtained is correct.
Evaluate ![]() using the integration-by-parts formula with
using the integration-by-parts formula with ![]() and
and ![]()
Answer
![]()
Hint
Find ![]() and
and ![]() and use the previous example as a guide.
and use the previous example as a guide.
The natural question to ask at this point is: How do we know how to choose ![]() and
and ![]() Sometimes it is a matter of trial and error; however, the acronym LIATE can often help to take some of the guesswork out of our choices. This acronym stands for Logarithmic Functions, Inverse Trigonometric Functions, Algebraic Functions, Trigonometric Functions, and Exponential Functions. This mnemonic serves as an aid in determining an appropriate choice for
Sometimes it is a matter of trial and error; however, the acronym LIATE can often help to take some of the guesswork out of our choices. This acronym stands for Logarithmic Functions, Inverse Trigonometric Functions, Algebraic Functions, Trigonometric Functions, and Exponential Functions. This mnemonic serves as an aid in determining an appropriate choice for ![]()
The type of function in the integral that appears first in the list should be our first choice of ![]() For example, if an integral contains a logarithmic function and an algebraic function, we should choose
For example, if an integral contains a logarithmic function and an algebraic function, we should choose ![]() to be the logarithmic function, because L comes before A in LIATE. The integral in
to be the logarithmic function, because L comes before A in LIATE. The integral in ![]() has a trigonometric function
has a trigonometric function ![]() and an algebraic function
and an algebraic function ![]() Because A comes before T in LIATE, we chose
Because A comes before T in LIATE, we chose ![]() to be the algebraic function. When we have chosen
to be the algebraic function. When we have chosen ![]()
![]() is selected to be the remaining part of the function to be integrated, together with
is selected to be the remaining part of the function to be integrated, together with ![]()
Why does this mnemonic work? Remember that whatever we pick to be ![]() must be something we can integrate. Since we do not have integration formulas that allow us to integrate simple logarithmic functions and inverse trigonometric functions, it makes sense that they should not be chosen as values for
must be something we can integrate. Since we do not have integration formulas that allow us to integrate simple logarithmic functions and inverse trigonometric functions, it makes sense that they should not be chosen as values for ![]() Consequently, they should be at the head of the list as choices for
Consequently, they should be at the head of the list as choices for ![]() Thus, we put LI at the beginning of the mnemonic. (We could just as easily have started with IL, since these two types of functions won’t appear together in an integration-by-parts problem.) The exponential and trigonometric functions are at the end of our list because they are fairly easy to integrate and make good choices for
Thus, we put LI at the beginning of the mnemonic. (We could just as easily have started with IL, since these two types of functions won’t appear together in an integration-by-parts problem.) The exponential and trigonometric functions are at the end of our list because they are fairly easy to integrate and make good choices for ![]() Thus, we have TE at the end of our mnemonic. (We could just as easily have used ET at the end, since when these types of functions appear together it usually doesn’t really matter which one is
Thus, we have TE at the end of our mnemonic. (We could just as easily have used ET at the end, since when these types of functions appear together it usually doesn’t really matter which one is ![]() and which one is
and which one is ![]() ) Algebraic functions are generally easy both to integrate and to differentiate, and they come in the middle of the mnemonic.
) Algebraic functions are generally easy both to integrate and to differentiate, and they come in the middle of the mnemonic.
Using Integration by Parts
Evaluate ![]()
Solution
Begin by rewriting the integral:
![]()
Since this integral contains the algebraic function ![]() and the logarithmic function
and the logarithmic function ![]() choose
choose ![]() since L comes before A in LIATE. After we have chosen
since L comes before A in LIATE. After we have chosen ![]() we must choose
we must choose ![]()
Next, since ![]() we have
we have ![]() Also,
Also, ![]() and so we take
and so we take ![]() . Summarizing,
. Summarizing,
![Rendered by QuickLaTeX.com \ds \begin{array}{ccccccc}\hfill u&\ds =\hfill &\ds \text{ln}\phantom{\rule{0.1em}{0ex}}(x)\hfill &\ds &\ds \hfill dv&\ds =\hfill &\ds {x}^{-3}\,dx \hfill \\[3mm]\ds \hfill du&\ds =\hfill &\ds \frac{1}{x}\,dx \hfill &\ds &\ds \hfill v&\ds =\hfill &\ds -\frac{1}{2}{x}^{-2}.\hfill \end{array}](https://pressbooks.openedmb.ca/app/uploads/quicklatex/quicklatex.com-8ec1add0bae676f8797814d97135f950_l3.png)
Substituting into (*) gives
![Rendered by QuickLaTeX.com \ds \begin{array}{ccccc}\hfill \ds\int \frac{\text{ln}\phantom{\rule{0.1em}{0ex}}(x)}{{x}^{3}}\,dx &\ds ={\int }^{\text{}}{x}^{-3}\text{ln}\phantom{\rule{0.1em}{0ex}}(x)\phantom{\rule{0.2em}{0ex}}\,dx \hfill\\[5mm]&\ds=\left(\text{ln}\phantom{\rule{0.1em}{0ex}}(x)\right)\left( - \phantom{\rule{0.2em}{0ex}}\frac{1}{2}{x}^{-2}\right)-{\int }^{\text{}}\left( - \phantom{\rule{0.2em}{0ex}}\frac{1}{2}{x}^{-2}\right)\left(\frac{1}{x}\,dx \right)\hfill &\ds &\ds &\ds \\[5mm]\ds &\ds =-\frac{1}{2}{x}^{-2}\text{ln}\phantom{\rule{0.1em}{0ex}}(x)+{\int }^{\text{}}\frac{1}{2}{x}^{-3}\,dx \hfill &\ds &\ds &\ds \text{simplify}\hfill \\[5mm]\ds &\ds =-\frac{1}{2}{x}^{-2}\text{ln}\phantom{\rule{0.1em}{0ex}}(x)-\frac{1}{4}{x}^{-2}+C.\hfill &\ds &\ds &\ds \text{integrate}\hfill \end{array}](https://pressbooks.openedmb.ca/app/uploads/quicklatex/quicklatex.com-d5c84a270d63e9d75048a638ee968db1_l3.png)
Evaluate ![]()
Answer
![]()
Hint
Use ![]() and
and ![]()
In some cases, as in the next two examples, it may be necessary to apply integration by parts more than once.
Applying Integration by Parts More Than Once
Evaluate ![]()
Solution
Using LIATE, choose ![]() and
and ![]() Thus,
Thus, ![]() and
and ![]() which means we can take
which means we can take ![]() . Therefore,
. Therefore,
![Rendered by QuickLaTeX.com \ds \begin{array}{ccccccc}\hfill u&\ds =\hfill &\ds {x}^{2}\hfill &\ds &\ds \hfill dv&\ds =\hfill &\ds {e}^{3x}\,dx \hfill \\[3mm]\ds \hfill du&\ds =\hfill &\ds 2x\phantom{\rule{0.2em}{0ex}}\,dx \hfill &\ds &\ds \hfill v&\ds =\hfill &\ds \frac{1}{3}{e}^{3x}.\hfill \end{array}](https://pressbooks.openedmb.ca/app/uploads/quicklatex/quicklatex.com-61d231257b8b0b25df0b4a99ef8e5bad_l3.png)
Substituting into (*) produces
![]()
We still cannot integrate ![]() directly, but the integral now has a lower power on
directly, but the integral now has a lower power on ![]() We can evaluate this new integral by using integration by parts again. To do this, choose
We can evaluate this new integral by using integration by parts again. To do this, choose ![]() and
and ![]() Thus,
Thus, ![]() and
and ![]() which means we can take
which means we can take ![]() . Now we have
. Now we have
![Rendered by QuickLaTeX.com \ds \begin{array}{ccccccc}\hfill u&\ds =\hfill &\ds x\hfill &\ds &\ds \hfill dv&\ds =\hfill &\ds \frac{2}{3}{e}^{3x}\,dx \hfill \\[3mm]\ds \hfill du&\ds =\hfill &\ds \,dx \hfill &\ds &\ds \hfill v&\ds =\hfill &\ds \frac{2}{9}{e}^{3x}.\hfill \end{array}](https://pressbooks.openedmb.ca/app/uploads/quicklatex/quicklatex.com-c2690bec28b78a57e24d4a7a8df9546a_l3.png)
Going back to the previous equation and using (*) yields
![Rendered by QuickLaTeX.com \ds\begin{array}{ll} \ds{\int }^{\text{}}{x}^{2}{e}^{3x}\,dx &=\ds\frac{1}{3}{x}^{2}{e}^{3x}-\int \frac{2}{3}x{e}^{3x}\,dx \hfill\\[5mm]&=\ds\frac{1}{3}{x}^{2}{e}^{3x}-\left(\frac{2}{9}x{e}^{3x}-{\int }^{\text{}}\frac{2}{9}{e}^{3x}\,dx \right).\hfill\end{array}](https://pressbooks.openedmb.ca/app/uploads/quicklatex/quicklatex.com-b475d9bc381ed76da2adbccb406313db_l3.png)
After evaluating the last integral and simplifying, we obtain
![]()
Applying Integration by Parts When LIATE Doesn’t Quite Work
Evaluate ![]()
Solution
If we use a strict interpretation of the mnemonic LIATE to make our choice of ![]() we end up with
we end up with ![]() and
and ![]() Unfortunately, this choice won’t work because we are unable to evaluate
Unfortunately, this choice won’t work because we are unable to evaluate ![]() However, since we can evaluate
However, since we can evaluate ![]() , we can try choosing
, we can try choosing ![]() and
and ![]() We then have
We then have
![Rendered by QuickLaTeX.com \begin{array}{ll}\ds\int t e^{t^2}\,dt&\ds=\int e^{t^2}\frac12 (2t)\,dt=\frac12\int e^{t^2}(t^2)'\, dt\hfill\\[5mm]&\ds=\frac12\int e^w dw=\frac12e^w+C=\frac12e^{t^2}+C,\end{array}](https://pressbooks.openedmb.ca/app/uploads/quicklatex/quicklatex.com-46c82401fe46a285e058038b70a51429_l3.png)
and so we can take ![]()
With these choices we have
![Rendered by QuickLaTeX.com \ds \begin{array}{ccccccc}\hfill u&\ds =\hfill &\ds {t}^{2}\hfill &\ds &\ds \hfill dv&\ds =\hfill &\ds t{e}^{{t}^{2}}dt\hfill \\[3mm]\ds \hfill du&\ds =\hfill &\ds 2t\phantom{\rule{0.2em}{0ex}}dt\hfill &\ds &\ds \hfill v&\ds =\hfill &\ds \frac{1}{2}{e}^{{t}^{2}}.\hfill \end{array}](https://pressbooks.openedmb.ca/app/uploads/quicklatex/quicklatex.com-4db403ae35fceef28b8de728572c1d6a_l3.png)
Thus, we obtain
![Rendered by QuickLaTeX.com \ds \begin{array}{cc}\ds {\int }^{\phantom{\rule{0.2em}{0ex}}}{t}^{3}{e}^{{t}^{2}}dt\hfill &\ds ={t}^{2}\cdot\frac{1}{2}e^{{t}^{2}}-\int \frac{1}{2}{e}^{{t}^{2}}2tdt\hfill \\[5mm]\ds \hfill &\ds =\frac{1}{2}{t}^{2}{e}^{{t}^{2}}-\frac{1}{2}{e}^{{t}^{2}}+C.\hfill \end{array}](https://pressbooks.openedmb.ca/app/uploads/quicklatex/quicklatex.com-0dedf62697cd4c6106783d08917d27ac_l3.png)
Applying Integration by Parts More Than Once
Evaluate ![]()
Solution
This integral appears to have only one function—namely, ![]() —however, we can always use the constant function 1 as the other function. In this example, let’s choose
—however, we can always use the constant function 1 as the other function. In this example, let’s choose ![]() and
and ![]() (The decision to use
(The decision to use ![]() is easy. We can’t choose
is easy. We can’t choose ![]() because if we could integrate it, we wouldn’t be using integration by parts in the first place!) Consequently,
because if we could integrate it, we wouldn’t be using integration by parts in the first place!) Consequently, ![]() and we can take
and we can take![]() as an antiderivative of 1. After applying integration by parts to the integral and simplifying, we obtain
as an antiderivative of 1. After applying integration by parts to the integral and simplifying, we obtain
Unfortunately, this process leaves us with a new integral that is very similar to the original. However, let’s see what happens when we apply integration by parts again. This time let’s choose ![]() and
and ![]() making
making ![]() and, again,
and, again, ![]() Substituting, we have
Substituting, we have
After simplifying, we obtain
The last integral is now the same as the original. It may seem that we have simply gone in a circle, but now we can actually evaluate the integral. To see how to do this more clearly, let ![]() be a particular antiderivative of
be a particular antiderivative of ![]() . Substituting
. Substituting ![]() instead of
instead of ![]() into the above equality, we obtain the following equation for
into the above equality, we obtain the following equation for ![]() .
.
![]()
To find ![]() , add
, add ![]() to both sides of the equation:
to both sides of the equation:
![]()
and then divide by 2
![]()
Since ![]() was a particular antiderivative of
was a particular antiderivative of ![]() , we have
, we have
![]()
where ![]() is an arbitrary constant.
is an arbitrary constant.
Analysis
If this method feels a little strange at first, we can check the answer by differentiation:
![Rendered by QuickLaTeX.com \ds \begin{array}{c}\ds\frac{d}{dx }\left(\frac{1}{2}x\phantom{\rule{0.1em}{0ex}}\text{sin}\left(\text{ln}\phantom{\rule{0.1em}{0ex}}(x)\right)-\frac{1}{2}x\phantom{\rule{0.1em}{0ex}}\text{cos}\left(\text{ln}\phantom{\rule{0.1em}{0ex}}(x)\right)\right)=\hfill\\[5mm]\ds =\frac{1}{2}\left(\text{sin}\left(\text{ln}\phantom{\rule{0.1em}{0ex}}(x)\right)\right)+\text{cos}\left(\text{ln}\phantom{\rule{0.1em}{0ex}}(x)\right)\cdot \frac{1}{x}\cdot \frac{1}{2}x-\left(\frac{1}{2}\text{cos}\left(\text{ln}\phantom{\rule{0.1em}{0ex}}(x)\right)-\text{sin}\left(\text{ln}\phantom{\rule{0.1em}{0ex}}(x)\right)\cdot \frac{1}{x}\cdot \frac{1}{2}x\right)\hfill \\[5mm]\ds =\text{sin}\left(\text{ln}\phantom{\rule{0.1em}{0ex}}(x)\right).\hfill \end{array}](https://pressbooks.openedmb.ca/app/uploads/quicklatex/quicklatex.com-f59d6fe773babe956527c9464188e2e4_l3.png)
Evaluate ![]()
Answer
![]()
Hint
This is similar to ![]() from one of the previous examples.
from one of the previous examples.
Integration by Parts for Definite Integrals
Now that we have used integration by parts successfully to evaluate indefinite integrals, we turn our attention to definite integrals. The integration technique is really the same, only we add a step to evaluate the integral at the upper and lower limits of integration.
Integration by Parts for Definite Integrals
Let ![]() and
and ![]() be functions with continuous derivatives on
be functions with continuous derivatives on ![]() Then
Then
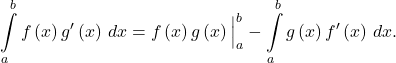
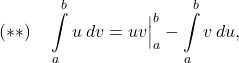
Finding the Area of a Region
Find the area of the region bounded above by the graph of ![]() and below by the x-axis over the interval
and below by the x-axis over the interval ![]()
Solution
This region is shown in Figure 1 below. To find the area, we must evaluate 
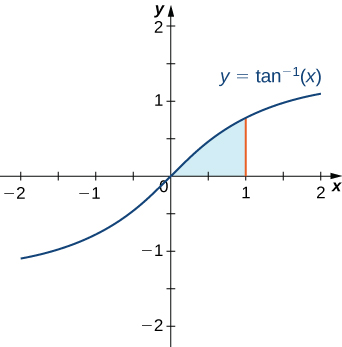
For this integral, let’s choose ![]() and
and ![]() thereby making
thereby making ![]() and
and ![]() After applying the integration-by-parts formula (**) for definite integrals, we obtain
After applying the integration-by-parts formula (**) for definite integrals, we obtain

We use a substitution of ![]() to evaluate
to evaluate 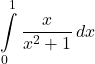 . We have that
. We have that ![]() , and hence
, and hence ![]() . Also, when
. Also, when ![]() ,
, ![]() , and when
, and when ![]() ,
, ![]() . It follows that
. It follows that
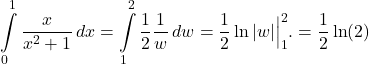
Therefore,
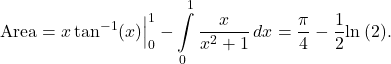
Finding a Volume of Revolution
Find the volume of the solid obtained by revolving the region bounded by the graph of ![]() the x-axis, the y-axis, and the line
the x-axis, the y-axis, and the line ![]() about the y-axis.
about the y-axis.
Solution
We use cylindrical shells method to solve this problem. Begin by sketching the region to be revolved, along with a typical rectangle (see the following graph).
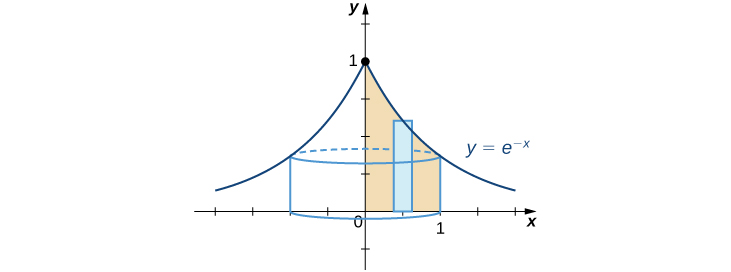
According to the formula, we must evaluate 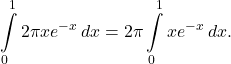
To do this, let ![]() and
and ![]() These choices lead to
These choices lead to ![]() and
and ![]() as an antiderivative of
as an antiderivative of ![]() . Using integration by parts, we obtain
. Using integration by parts, we obtain
![Rendered by QuickLaTeX.com \ds \begin{array}{cccc}\hfill \text{Volume}&\ds =2\pi \underset{0}{\overset{1}{\int }}x{e}^{ - x}\,dx =2\pi \left( - x{e}^{ - x}{}\Big|_{0}^{1}+\underset{0}{\overset{1}{\int }}{e}^{ - x}\,dx \right)\hfill &\ds &\ds \hfill \\[5mm]\ds &\ds =-2\pi x{e}^{ - x}{}\Big|_{0}^{1}-2\pi {e}^{ - x}{}\Big|_{0}^{1}\hfill &\ds &\ds \hfill \\[5mm]\ds &\ds =2\pi -\frac{4\pi }{e}.\hfill &\ds &\ds \hfill \end{array}](https://pressbooks.openedmb.ca/app/uploads/quicklatex/quicklatex.com-a0115fe8d903a26ca6b7efd8ba84e21f_l3.png)
Evaluate 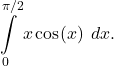
Answer
![]()
Hint
Use integration by parts with ![]() and
and ![]()
Key Concepts
- The integration-by-parts formula allows the exchange of one integral for another, possibly easier, integral.
- Integration by parts applies to both definite and indefinite integrals.
Key Equations
- Integration by parts formula
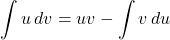
- Integration by parts for definite integrals
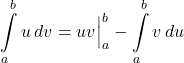
Exercises
In using the technique of integration by parts, you must carefully choose which expression is u. For each of the following exercises, use the guidelines in this section to choose u. Do not evaluate the integrals.
1. ![]()
Answer
![]()
2. ![]()
3. ![]()
Answer
![]()
4. ![]()
5. ![]()
Answer
![]() or
or ![]() ; this example requires two applications of integration by parts, and it is important to choose
; this example requires two applications of integration by parts, and it is important to choose ![]() in the same way in both of them.
in the same way in both of them.
For the following exercises, evaluate the integral using integration by parts.
6. ![]()
7. ![]()
(Hint: ![]() is equivalent to
is equivalent to ![]() )
)
Answer
![]()
8. ![]()
9. ![]()
Answer
![]()
10. ![]()
11. ![]()
Answer
![]()
12. ![]()
13. ![]()
Answer
![]()
14. ![]()
15. ![]()
Answer
![]()
16. ![]()
17. ![]()
Answer
![]()
18. ![]()
19. ![]()
Answer
![]()
20. ![]()
21. ![]()
Answer
![]()
22. ![]()
23. ![]()
Answer
![]()
24. ![]()
(Hint: you can simplify the integrand using properties of logarithms.)
25. ![]()
Answer
![]()
26. ![]()
27. ![]()
Answer
![]()
28. ![]()
29. ![]()
Answer
![]()
30. ![]()
31. ![]()
Answer
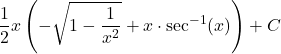
32. ![]()
33. ![]()
Answer
![]()
For the following exercises, compute the given definite integral using integration by parts.
34. 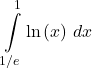
35. 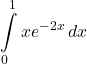
Answer
![]()
36. 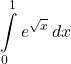
(Hint: make a substitution ![]() )
)
37. 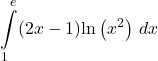
Answer
![]()
38. 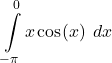
39. 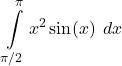
Answer
![]()
40. 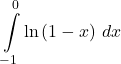
41. 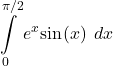
Answer
![]()
42. 
43. Evaluate 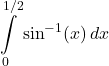
Answer
![]()
Assume that n is a positive integer. Derive the following formulas using integration by parts. These formulas are called reduction formulas because the exponent in the x term has been reduced by one in each case.
44. ![]()
45. ![]()
Answer
Take ![]() and
and ![]() .
.
46. ![]()
47. Integrate ![]() using two methods:
using two methods:
- Using parts, letting
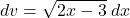
- Substitution, letting
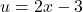
Answer
![]()
State whether you would use integration by parts to evaluate the integral. If so, identify u and dv. If not, describe the technique you would use. You don’t have to evaluate the integral.
48. ![]()
49. ![]()
Answer
No need to use integration by parts. Make a substituion ![]() .
.
50. ![]()
51. ![]()
Answer
No need to use integration by parts. Make a substitution ![]() .
.
52. ![]()
53. ![]()
Answer
No need to use integration by parts. Make a substitution ![]() .
.
For the following exercises, sketch the region bounded by the given curves and find its area using integration by parts.
54. ![]()
55*. ![]()
(Hint: take ![]() and
and ![]() .)
.)
Answer
![]()
56. ![]()
57. ![]()
Answer
![]()
58. ![]()
For the following exercises, find the volume generated by rotating the region bounded by the given curves about the specified axes.
59. ![]() about the x-axis.
about the x-axis.
Answer
![]()
60. ![]() about the y-axis
about the y-axis
61. ![]() ,
, ![]() about
about ![]()
Answer
![]()
62. ![]() about the x-axis.
about the x-axis.
63. ![]() about the y-axis.
about the y-axis.
Answer
![]()
64. A particle moving along a straight line has a velocity of ![]() after t sec. How far does it travel in the first 2 sec?
after t sec. How far does it travel in the first 2 sec?
Glossary
- integration by parts
- a technique of integration that allows the exchange of one integral for another using the formula