Chapter 5: Work, Power, and Energy

Conceptual Questions

5.1 Work

  1. Give an example of something we think of as work in everyday circumstances that is not work in the scientific sense. Is energy transferred or changed in form in your example? If so, explain how this is accomplished without doing work.
  2. Give an example of a situation in which there is a force and a displacement, but the force does no work. Explain why it does no work.
  3. Describe a situation in which a force is exerted for a long time but does no work. Explain.
  4. *A body moves in a circle at constant speed. Does the centripetal force that accelerates the body do any work? Explain.
  5. *Suppose you throw a ball upward and catch it when it returns at the same height. How much work does the gravitational force do on the ball over its entire trip?
  6. *Why is it more difficult to do sit-ups while on a slant board than on a horizontal surface? (See below.)
    Illustrations of a person doing sit ups while on a slanted board (with feet above the head) and of a person doing sit ups while on a horizontal surface.
  7. *As a young man, Tarzan climbed up a vine to reach his tree house. As he got older, he decided to build and use a staircase instead. Since the work of the gravitational force mg is path independent, what did the King of the Apes gain in using stairs?

5.2 Kinetic Energy and the Work-Energy Theorem

  1. The kinetic energy of a system must always be positive or zero. Explain whether this is true for the potential energy of a system. 
  2. The person in the figure does work on the lawn mower. Under what conditions would the mower gain energy? Under what conditions would it lose energy?A person pushing a lawn mower with a force F. Force is represented by a vector making an angle theta below the horizontal and distance moved by the mover is represented by vector d. The component of vector F along vector d is F cosine theta. Work done by the person, W, is equal to F d cosine theta.Solution: The mower would gain energy if −90°<θ<90°. It would lose the energy if 90°<θ<270°. The mower may also lose energy due to friction with the grass while pushing; however, we are not concerned with that energy loss for this problem.
  3. Work done on a system puts energy into it. Work done by a system removes energy from it. Give an example for each statement.
  4. When solving for speed in a problem, we kept only the positive root. Why?
  5. *Suppose you are jogging at constant velocity. Are you doing any work on the environment and vice versa?
  6. *The kinetic energy of a system must always be positive or zero. Explain whether this is true for the potential energy of a system.
  7. *A person drops a pebble of mass m1 from a height h, and it hits the floor with kinetic energy K. The person drops another pebble of mass m2 from a height of 2h. How do the masses of the pebbles compare?

5.3 Gravitational Potential Energy

  1. In the roller coaster figure from the chapter (same as below), we calculated the final speed of a roller coaster that descended 20 m in height and had an initial speed of 5 m/s downhill. Suppose the roller coaster had had an initial speed of 5 m/s uphill instead, and it coasted uphill, stopped, and then rolled back down to a final point 20 m below the start. We would find in that case that its final speed is the same as its initial speed. Explain in terms of conservation of energy.
    negligible friction) if its initial speed is 5.00 m/s?

    A roller coaster track is shown with a car about to go downhill. The initial height of the roller coaster car on the track is twenty-five meters from the lowest part of the track and its speed v sub zero is equal to zero. The roller coaster’s height from the level part of the track is twenty meters. The finish point of the car is on the level part of the track and the speed at that point is unknown.
  2. Does the work you do on a book when you lift it onto a shelf depend on the path taken? On the time taken? On the height of the shelf? On the mass of the book?
  3. *The kinetic energy of a system must always be positive or zero. Explain whether this is true for the potential energy of a system.
    Solution: The potential energy of a system can be negative because its value is relative to a defined point.
  4. *The force exerted by a diving board is conservative, provided the internal friction is negligible. Assuming friction is negligible, describe changes in the potential energy of a diving board as a swimmer drives from it, starting just before the swimmer steps on the board until just after his feet leave it.
  5. *Describe the gravitational potential energy transfers and transformations for a javelin, starting from the point at which an athlete picks up the javelin and ending when the javelin is stuck into the ground after being thrown.
    Solution: If the reference point of the ground is zero gravitational potential energy, the javelin first increases its gravitational potential energy, followed by a decrease in its gravitational potential energy as it is thrown until it hits the ground. The overall change in gravitational potential energy of the javelin is zero unless the center of mass of the javelin is lower than from where it is initially thrown, and therefore would have slightly less gravitational potential energy.
  6. *A couple of soccer balls of equal mass are kicked off the ground at the same speed but at different angles. Soccer ball A is kicked off at an angle slightly above the horizontal, whereas ball B is kicked slightly below the vertical. How do each of the following compare for ball A and ball B?
    (a) The initial kinetic energy and
    (b) the change in gravitational potential energy from the ground to the highest point? If the energy in part (a) differs from part (b), explain why there is a difference between the two energies.
  7. A child jumps up and down on a trampoline, reaching a higher height after each bounce. Explain how the child can increase his maximum gravitational potential energy with each bounce.
    Solution: He puts energy into the system through his legs compressing and expanding.

5.4 Conservative Forces and Potential Energy

  1. What is a conservative force?
  2. The force exerted by a diving board is conservative, provided the internal friction is negligible. Assuming friction is negligible, describe changes in the potential energy of a diving board as a swimmer dives from it, starting just before the swimmer steps on the board until just after his feet leave it.
  3. Define mechanical energy. What is the relationship of mechanical energy to nonconservative forces? What happens to mechanical energy if only conservative forces act?
  4. What is the relationship of potential energy to conservative force?

5.5 Nonconservative Forces

  1. What is the physical meaning of a non-conservative force?
    Solution: A force that takes energy away from the system that can’t be recovered if we were to reverse the action
  2. Can a non-conservative force increase the mechanical energy of the system?
  3. Neglecting air resistance, how much would I have to raise the vertical height if I wanted to double the impact speed of a falling object?
    Solution: Four times the original height would double the impact speed

5.6 Conservation of Energy

  1. Consider the following scenario. A car for which friction is not negligible accelerates from rest down a hill, running out of gasoline after a short distance. The driver lets the car coast farther down the hill, then up and over a small crest. He then coasts down that hill into a gas station, where he brakes to a stop and fills the tank with gasoline. Identify the forms of energy the car has, and how they are changed and transferred in this series of events. 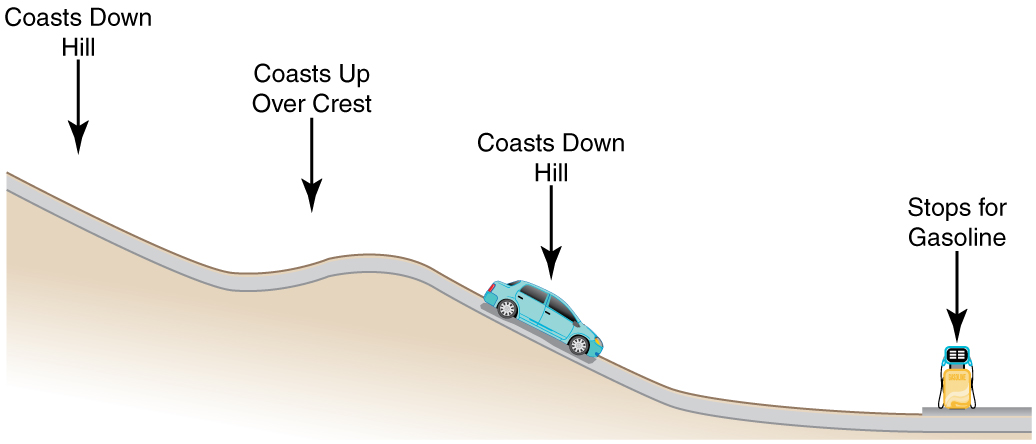(See figure below)A car coasting downhill, moving over a crest then again moving downhill and finally stopping at a gas station. Each of these positions is labeled with an arrow pointing downward.
  2. Describe the energy transfers and transformations for a javelin, starting from the point at which an athlete picks up the javelin and ending when the javelin is stuck into the ground after being thrown.
  3. Do devices with efficiencies of less than one violate the law of conservation of energy? Explain.
  4. List four different forms or types of energy. Give one example of a conversion from each of these forms to another form.
  5. List the energy conversions that occur when riding a bicycle.

5.7 Power

  1. Most electrical appliances are rated in watts. Does this rating depend on how long the appliance is on? (When off, it is a zero-watt device.) Explain in terms of the definition of power.
  2. Explain, in terms of the definition of power, why energy consumption is sometimes listed in kilowatt-hours rather than joules. What is the relationship between these two energy units?
  3. A spark of static electricity, such as that you might receive from a doorknob on a cold dry day, may carry a few hundred watts of power. Explain why you are not injured by such a spark.
  4. *Does the work done in lifting an object depend on how fast it is lifted? Does the power expended depend on how fast it is lifted?
  5. *Can the power expended by a force be negative?

5.8 Work, Energy, and Power in Humans

  1. Explain why it is easier to climb a mountain on a zigzag path rather than one straight up the side. Is your increase in gravitational potential energy the same in both cases? Is your energy consumption the same in both?
  2. Do you do work on the outside world when you rub your hands together to warm them? What is the efficiency of this activity?
  3. Shivering is an involuntary response to lowered body temperature. What is the efficiency of the body when shivering, and is this a desirable value?
  4. Discuss the relative effectiveness of dieting and exercise in losing weight, noting that most athletic activities consume food energy at a rate of 400 to 500 W, while a single cup of yogurt can contain 1360 kJ (325 kcal). Specifically, is it likely that exercise alone will be sufficient to lose weight? You may wish to consider that regular exercise may increase the metabolic rate, whereas protracted dieting may reduce it.

License

Icon for the Creative Commons Attribution 4.0 International License

Introduction to Biomechanics Copyright © 2022 by Rob Pryce is licensed under a Creative Commons Attribution 4.0 International License, except where otherwise noted.

Share This Book