Chapter 1: Introduction
1.2 Physical Quantities and Units
Authors: William Moebs, Samuel Ling, Jeff Sanny
Adapted by: Rob Pryce, Alix Blacklin
Learning Objectives
By the end of this section, you will be able to:
- Describe how SI base units are defined.
- Describe how derived units are created from base units.
- Express quantities given in SI units using metric prefixes
In order to communicate effectively about human movement we need a common framework for describe and measure movement. In biomechanics we’re primarily concerned with three basic properties related to movement. These are:
1) Mass: the amount of matter in an object; used to derive weight
2) Length: used to describe the distance between objects or points
3) Time: the duration between two or more events
These are referred to as physical quantities because they represent properties of an object or system that we can measure. We define physical quantities either by specifying how they are measured or by stating how they are calculated from other measurements. For example, we might define distance and time by specifying methods for measuring them, such as using a meter stick and a stopwatch. Then, we could calculate speed as distance divided by time.
Each of these physical quantities also needs a unit of measure – that is, a standardized way for representing the size or amount of the property. For example, the length of a running race which is a physical quantity, can be expressed in units of meters (for sprinters) or kilometers (for distance runners). Without these standardized units, it would be extremely difficult for people to communicate and compare measurements related to human movement (Figure 1.7)
In biomechanics we use one system to define physical quantities and units of measure: SI units (for the French Système International d’Unités), also known as the metric system. The SI system is also referred to as the kilogram-metres-second system (after the respective base units, see below). Virtually every country in the world now uses SI units as the standard and this is also the system agreed on by scientists and mathematicians in every field. So, being able to use SI units ensures that when we’re describing physical quantities in human movement – such as distance, speed, weight, or force – everyone in the field of biomechanics (across the entire world) knows what we’re talking about.
However, a common source of confusion (and consternation) when dealing with physical quantities is that we often find ourselves using non-SI units when communicating with the general public or other ‘non-biomechanics’ knowledge users. Most commonly, this comes in the form of English units (also known as the customary or imperial system). English units were historically used in nations once ruled by the British Empire and are still widely used in the United States and by many members of the general public (including even ourselves). For example, undoubtedly, all of us have discussed things like speed using miles per hour or weight using pounds at some point in our life.
Now, the most stringent users of the SI system might simply refuse to communicate in units other than SI, however a much more reasonable (and useful) approach is to be able to communicate in both SI and English units, or even in other units that might be more familiar to our intended audience. Arguably, being able to communicate about human movement using units that are meaningful and appropriate to our audience is as important as having a consistent standard of measurement. So, every good student of biomechanics must not only be familiar with SI units, but also be able to convert back and forth between SI, English and other non-standard units of measure. These conversions are covered at the end of this chapter.
SI Units: Base and Derived Units
In any system of units, the units for some physical quantities must be defined through a measurement process. These are called the base quantities for that system and their units are the system’s base units. In biomechanics, the most common base units are the kilogram (mass), metre (length) and second (time). All other physical quantities in biomechanics can then be expressed as mathematical combinations of these base quantities. Those are then known as a derived quantities, with units of measure called derived units.
Table 1.1 lists these seven base quantities and units that make up the SI/metric system. The vast majority of biomechanics (and the entirety of this book) is concerned with the first three quantities, however other base quantities and units may be encountered when applying biomechanics to other aspects of human movement (e.g. human movement in different environmental conditions, such as temperature or light variations)
Table 1.1 SI Base Quantities and Their Units of Measure
| Base Quantity | Unit (abbreviation) |
| Length | meter (m) |
| Mass | kilogram (kg) |
| Time | second (s) |
| Electrical Current | ampere (A) |
| Temperature | kelvin (k) |
| Amount of substance | mole (mol) |
| Luminous intensity | candela (cd) |
You are probably already familiar with some derived quantities that can be formed from the base quantities in Table 1.1. For example, speed is length per time; so in terms of SI base units, we should measure it in meters per second (m/s). Density is mass per volume, which is expressed in terms of SI base units such as kilograms per cubic meter (kg/m3). In biomechanics we can use density, which is mass divided by volume (kg/m3), to estimate body composition (the relative amount of muscle, fat and bone in the human body). Even angles, which are the most common way of describing the position of body segments (e.g., the angle of the arm relative to the trunk), are derived quantities. They can be calculated using arc lengths and radius of a circle – in fact, that is how the ‘radian’ is defined (the SI unit for angle) One of the most important derived units in biomechanics is the Newton (N), used to measure force, and is defined as the force needed to accelerate a mass of 1 kilogram by 1 metre/sec2.We will see many more examples throughout this text.
Units of Time, Length, and Mass: The Second, Meter, and Kilogram
In the field of biomechanics, nearly all all relevant physical quantities can be expressed in terms of the base units of length, mass, and time. Therefore, we now turn to a discussion of these three base units.
The second
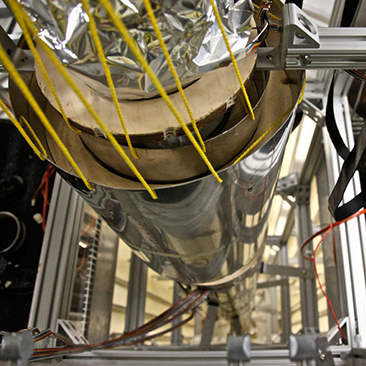
The SI unit for time, the second (abbreviated s), has a long history. For many years it was defined as 1/86,400 of a mean solar day. More recently, a new standard was adopted to gain greater accuracy and to define the second in terms of a nonvarying or constant physical phenomenon (because the solar day is getting longer as a result of the very gradual slowing of Earth’s rotation). Cesium atoms can be made to vibrate in a very steady way, and these vibrations can be readily observed and counted. In 1967, the second was redefined as the time required for 9,192,631,770 of these vibrations to occur (Figure 1.8). Note that this may seem like more precision than you would ever need, but it isn’t—GPSs rely on the precision of atomic clocks to be able to give precise estimates of your location on the surface of Earth, far from the satellites broadcasting their location. Many sports, such as cycling, soccer, and running, now use GPS to provide athletes with information about speed, distance and the route traveled.
The meter
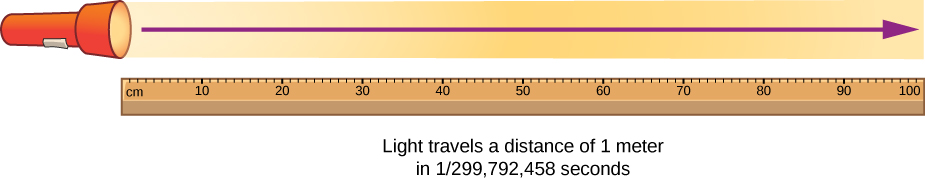
The SI unit for length is the meter (abbreviated m); its definition has also changed over time to become more precise. The meter was first defined in 1791 as 1/10,000,000 of the distance from the equator to the North Pole. This measurement was improved in 1889 by redefining the meter to be the distance between two engraved lines on a platinum–iridium bar now kept near Paris. By 1960, it had become possible to define the meter even more accurately in terms of the wavelength of light, so it was again redefined as 1,650,763.73 wavelengths of orange light emitted by krypton atoms. In 1983, the meter was given its current definition (in part for greater accuracy) as the distance light travels in a vacuum in 1/299,792,458 of a second (Figure 1.9). This change came after knowing the speed of light to be exactly 299,792,458 m/s.
The kilogram
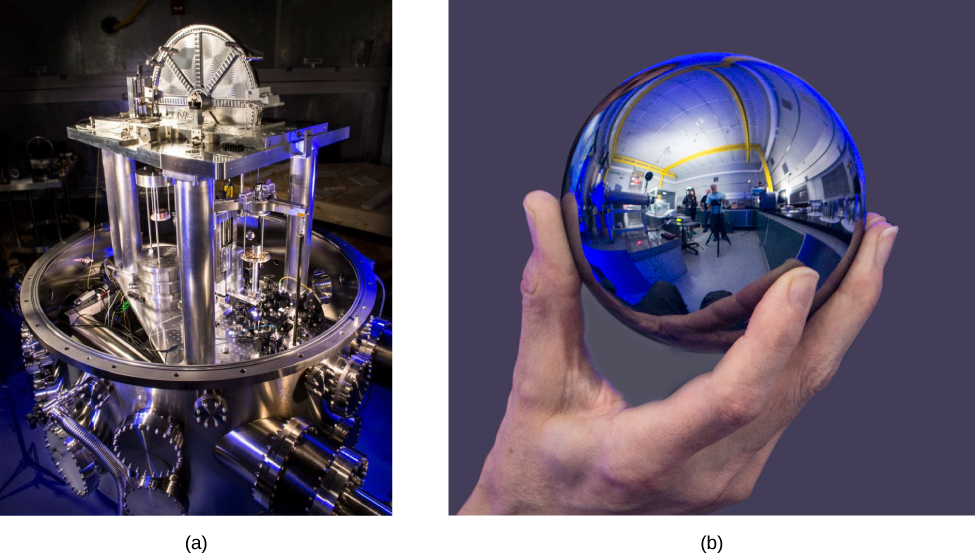
The SI unit for mass is the kilogram (abbreviated kg); From 1795–2018 it was defined to be the mass of a platinum–iridium cylinder kept with the old meter standard at the International Bureau of Weights and Measures near Paris. However, this cylinder has lost roughly 50 micrograms since it was created. Because this is the standard, this has shifted how we defined a kilogram. Therefore, a new definition was adopted in May 2019 based on the Planck constant and other constants in quantum mechanics which will never change in value. (quantum mechanics is an area of physics that describes how the smallest pieces of the universe work, but is not part of this textbook). The kilogram is measured on a Kibble balance (Figure 1.10). When a weight is placed on a Kibble balance, an electrical current is produced that is proportional to Planck’s constant. Since Planck’s constant is defined, the exact current measurements in the balance define the kilogram. You can explore the interesting history of mass standards at the website of the Physical Measurement Laboratory.
Metric Prefixes
SI units are part of the metric system, which is convenient for biomechanical calculations because the units are categorized by factors of 10. Table 1.2 lists the metric prefixes and symbols used to denote common factors of 10 in SI units used in biomechanics. For example, a centimeter is one-hundredth of a meter (in symbols, 1 cm = 10–2 m), a kilometer is a thousand meters (1 km = 103 m), and a millisecond is a thousandth of a second..
Table 1.2 Metric prefixes for powers of 10 and their symbols
| Prefix | Symbol | Meaning | Prefix | Symbol | Meaning |
| giga- | G | 109 | nano- | n | 10-9 |
| mega- | M | 106 | micro- | [latex]\mu[/latex] | 10-6 |
| kilo- | k | 103 | milli- | m | 10-3 |
| hecto- | h | 102 | centi- | c | 10-2 |
| deka- | da | 101 | deci- | d | 10-1 |
The only rule when using metric prefixes is that you cannot “double them up.” For example, if you have measurements in kilometers (1 km = 103 m), it is not proper to talk about hectodecameters, even though 102×101=103. So, when you’re adding prefixes to units of length (m), mass (g) or time (s), be sure to use only one prefix.
As we will see in the next section, one big advantage of using the metric system is that that conversions of units are simple, involving only powers of 10. For example, there are 100 cm in 1 m, 1000 m in 1 km, and so on. In nonmetric systems, such as the Imperial system of units, the relationships are not as simple—there are 12 in. in 1 ft, 5280 ft in 1 mi, and so on. Converting between metric and imperial units will be covered at the end of this section.
Another advantage of metric systems is that the same unit can be used over extremely large ranges of values simply by using the appropriate prefix. For example, we could use distances in meters for short-distance track and field events (e.g., 100m, 200m, 4000m), whereas distances in kilometers are more appropriate for long distance, endurance events (e.g., it is more appropriate to say a marathon is 42.2 km, rather than 42,195 m). . By comparison, in the metric system we might refer to short distance events using ‘yards’ and long distance events in miles (e.g., a marathon is 26.2 miles). However, yards and miles are two different units of measure, and unless you know how many yards are in a mile (1760), converting between the two is difficult and non-intuitive (never mind it is much harder to multiply and divide by 1760 compared to by 10). So, with the the metric system there is no need to invent new units for different applications. Instead, we can easily rescale the units using simple prefixes that relate to multiples of 10.
Example 1.1
Using Scientific Notation and Metric Prefixes in Biomechanics
Restate the mass 1,950,000 g and length 0.000065 m in:
a) scientific notation, and
b) using a metric prefix such that the resulting numerical value is bigger than one and less than 1000.
Strategy
Using scientific notation is one of the easiest ways to convert between different metric prefixes. It also allows us to write very large or small numbers more concisely. In order to convert between different metric prefixes in scientific notation we simply keep track of the number of powers of 10 in the numerical value and prefix, and ensure they sum to the same power of 10 as the original answer. The solutions below will demonstrate that process and also provide a refresher on scientific notation.
Solution a)
To convert a number to scientific notation we move the decimal place to the left or right to arrive at a number between 1 and 10. The number of places we move the decimal place corresponds to the power of 10 we will use and the direction (left or right) indicates whether the power of 10 is positive or negative. If we move the decimal place to the left the power of 10 is positive and if we move it to the right the power of 10 is negative.
For 1,950,000 we can first write the number without the commas and with the decimal place: 1950000.0 (note: in this case the decimal place is indicated for convenience, it does not imply a greater level of precision). Next, we can move the decimal 6 places to the left to arrive at 1.95 (a number between 1 and 10). Since the decimal moved 6 places to the left, the corresponding power of 10 is x 106. So, in scientific notation the answer is 1.95 x 106 g.
In the case of 0.000065 we follow the same process, but instead are moving the decimal place to the right 5 places, which will mean our power of 10 is negative. In scientific notation the answer is 6.5 x 10-5 m.
If you’d like a more in-depth refresher on scientific notation check out this webpage and lesson by Khan Academy
Solution b)
To express the number using a metric prefix we can look up the closest power of 10 in Table 1.2 and find the corresponding prefix.
For 1.95 x 106 g we can see that 106 corresponds to the prefix ‘mega’, or M. Since the prefix has the same powers of 10 as our scientific notation we can simply write the answer as 1.95 Mg.
For 6.5 x 10-5 m we can see that the two closest prefixes are ‘milli’, m, and ‘micro’ [latex]\mu[/latex] which correspond to 10-3 and 10-6 respectively. Using the prefix ‘milli’ we’d have 6.5 x 10-2 mm and for the prefix ‘micro’ we’d have 6.5 x 101 [latex]\mu{m}[/latex]. To arrive at the correct power of 10 we ensured the power of 10 in the prefix (-3 or -6) and the power of 10 remaining (-2 or 1) added up to the original power of 10 (-5). So, for ‘milli’ we’d have -3 + -2 = -5 and for ‘micro’ we’d have 1 + -6 = -5.
Since the problem asks us to select a prefix where the numerical value is above one and less than 1000, we’d convert back from scientific notation and select the appropriate metric prefix. Since 6.5 x 10-2 is equal to 0.065, it doesn’t appear the prefix ‘milli’ will be appropriate. However, 6.5 x 101 is equal to 65, which is between one and 1000. So our final answer is 65 [latex]\mu{m}[/latex].
Discussion
It is easy to make silly arithmetic errors when switching from one prefix to another, so it is always a good idea to check that our final answer matches the number we started with. An easy way to do this is follow the same process above and put both numbers in scientific notation and count powers of 10, including the ones hidden in prefixes. If we did not make a mistake, the powers of 10 should match up. In this problem, we started with 0.000065 m, which was 6.5 x 10-5 m. Our final answer in scientific notation was 6.5 x 101 [latex]\mu{m}[/latex]. Since ‘micro’ or [latex]\mu[/latex] has -6 powers of 10, we have 1 + -6 = -5 powers of 10 (which we already saw above). So, everything checks out.
If this mass or length arose from a calculation in a problem we were given, we would also want to check to determine whether numbers this large or small makes any sense in the context of the problem. For this Figure 1.11 below might be helpful.
Known ranges of length, mass, and time
Sometimes it is useful to have some context for the magnitude of different answers or values we encounter in biomechanics. Although most of the values we encounter in the study of human motion are constrained to a relatively small range of 1 x 10-3 to 1 x 106 across many of the base and derived quantities in the field, it is useful to consider the full range of known values for length, mass and time. This will not only give you a feeling for the range of possible numerical values in biomechanics, it will also help you detect incorrect answers that resulted from calculation errors (numbers that are far too large or too small to be realistic for the study of human movement. Figure 1.11 provides examples of the known range of values for length, mass and time.
Visit this site to explore interactively the vast range of length scales in our universe. Scroll down and up the scale to view hundreds of organisms and objects, and click on the individual objects to learn more about each one.
Check your Understanding
Restate [latex]4.79\,×\,{10}^{5}\text{kg}[/latex] using a metric prefix such that the resulting number is bigger than one but less than 1000.
Answer: [latex]4.79\,×\,{10}^{2}[/latex] Mg or 479 Mg

